Romps, Clausius-Clapeyron scaling of CAPE from analytical solutions to RCE, JAS, 2016
Paper
Description
Severe weather and lightning are both predicted to become more frequent with global warming (Seeley and Romps, 2015; Romps et al., 2014). These predictions are based on taking successful proxies for severe weather and lightning and applying them to future climate simulations. Those proxies depend on convective available potential energy (CAPE), which the GCMs predict will increase exponentially -- at a rate roughly equivalent to Clausius-Clapeyron scaling -- and these increases lead to the prediction of large increases in the frequency of severe weather and lightning.
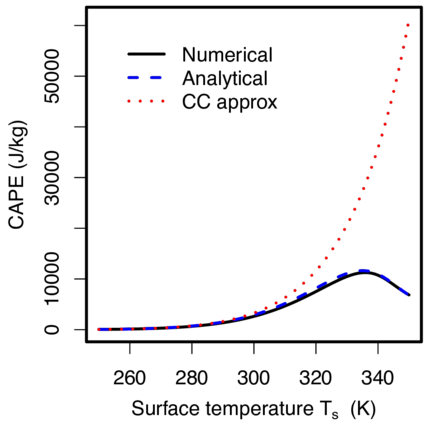
Despite the significant societal implications of increases in CAPE, there has been no successful theory for why CAPE takes the values it does in the current climate, or why it increases with warming. Only in the past few years has research identified the physical processes that set CAPE in the current climate (Singh and O'Gorman, 2013) and the processes that cause CAPE to increase with warming (Seeley and Romps, 2016). But, the question remains: Why does the increase in CAPE match Clausius-Clapeyron scaling so well? This paper derives the first analytical solutions to a moist-convective atmosphere. From these solutions, we obtain an analytical solution for CAPE, which explains why CAPE exhibits CC scaling at surface temperatures below about 310 K. Above 310 K, CAPE ceases to increase in proportion to near-surface saturated water-vapor mixing ratio and even decreases with warming above a surface temperature of about 325 K. The reasons for this behavior are also explained.
The dependence of CAPE on surface temperature Ts for radiative-convective equilibrium (RCE). (black solid) Numerical calculation of CAPE, which exhibits Clausius-Clapeyron (CC) scaling at surface temperatures at and below about 310 K. (blue dashed) The full analytical expression for CAPE, which matches the numerical calculation for all surface temperatures. (red dotted) The approximate analytical expression for CAPE, which incorrectly predicts CC scaling at very warm temperatures.